N.2.5 Types of division problems, and models for division.
Division is the opposite of multiplication, so one defintition for division is the fact-family form of division:
An example of a multiplication fact family is:
| 3 x 4 = 12 | 4 x 3 = 12 | 12 ÷ 3 = 4 | 12 ÷ 4 = 3 |
So a way to define division is to say that
N ÷ M = L and N ÷ L = M if (and only if) L x M = N (but we have to be careful about what to do when M or L is 0--see next section)
Notice that in the fact family has two members that are division statements, so if we use the usual model of multiplication with my example :
I have 4 groups, with 3 things in each group. How many things are there altogether? (4 x 3 = 12)
I can make two different kinds of division questions to go with it: one where I know the number of groups, and one where I know the number of things in each group. These different kinds of questions are the two most important models for division. Here are examples using the same situation as in my multiplication problem
Partitive division: I have 12 things, and I put them in 4 equal groups. How many are in each group? (12 ÷ 4 = 3)
* Measurement division: I have 12 things, and I put them in groups of 3. How many are in each group? (12 ÷ 3 = 4)
**** Warning! Every time I teach this class, I ask students to write measurement division word problems as part of their homework assignment, and every time a lot of students write partitive division problems that have a measurement context (for example: John has 12 inches of bubble tape, which he shares among 3 people. How much bubble tape does each person get?--You are measuring in inches, so the context is measuring, but you know how many groups (people) not how many in each group (number of inches each person gets) so the model is partitive). This is tricky, because there are two different ways of using the word measurement. If you don't know which I meant, ASK!
Children can solve both kinds of division problems by direct modeling, and these are reasonable problems for many children as early as K-1 if they are using direct modeling to find the solution.
Measurement problems are direct modeled by making groups. The problem:
Johnny has 12 cupcakes. He puts 3 cupcakes on each plate. How many plates does he fill?
would be solved by repeatedly taking groups of 3 objects from a pile of 12 objects:
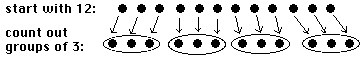
A counting strategy for doing the same thing would be to repeatedly subtract 3 from 12, and count how many times you subtracted:
12-3=9
9-3=6
6-3=3
3-3=0
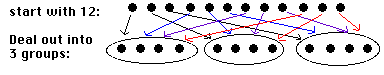
Partition problems are direct modeled by dealing out objects into groups. The problem:
Johnny has 12 cupcakes. He puts the cupcakes into 3 boxes. How many cupcakes are in each box?
would be solved by giving one cupcake to each of 3 boxes. Note that if children are asked to solve a partition problem by direct modeling with objects, they will often use objects to represent the boxes as well as the objects that represent the cupcakes. If the problems are asked about numbers that are a reasonable size, children usually do not get confused between their cupcakes and their boxes.
Here is a picture representing this direct modeling strategy. You should read along the top line of objects from left to right to see what children do and in what order. I have represented my boxes with circles, and not with the same dots I am using for cupcakes, because that is a little easier to follow on paper:
This model is harder to translate into a counting strategy, though it can be done by the same counting strategy as illustrated for measurement division.
Because both of these models correspond directly to the multiplication model, it is easy to see how they can be solved by using missing number multiplication problems: _ x 3 = 12. This is the best strategy for children who know their multiplication facts.
Notice that while both types of problems correspond well with a missing number multiplication strategy, measurement division leads much more naturally to understanding the repeated subtraction strategy for solving division problems. Some teachers (and textbooks) seem to prefer partition division problems to measurement division, but because students need to understand both the repeated subtraction and missing number multiplication strategies for division in order to understand the long division algorithm, it is important for children to also have experience with measurement division problems.

