

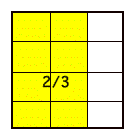
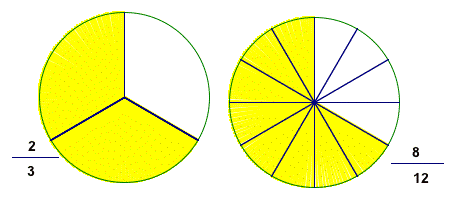
Now fold it in fourths going the other way.
When you open it up, you will find that you have 8/12 little rectangles shaded. This is a very tactile way of showing that 2/3 = 8/12.
One of the most significant topics in fractions is the question of fraction equivalence. Equivalence is the key concept on which our methods for adding, subtracting and comparing fractions are based. Rectangular (square or rectangle) models of fractions are the most powerful for showing equivalence. This is often done by paper folding:
 | Take a piece of paper, and fold it in thirds, and open it back up. |
 | Shade in 2 of the thirds, and label it 2/3. |
 | Now fold it in fourths going the other way. When you open it up, you will find that you have 8/12 little rectangles shaded. This is a very tactile way of showing that 2/3 = 8/12. |
This works so nicely because a rectangle has two dimensions you can fold on, not just one. Use one direction for your more reduced fraction, and in the other direction you fold into as many pieces as you would multiply numerator and denominator by. This method also leads nicely to the multiply numerator and denominator rule thusly:
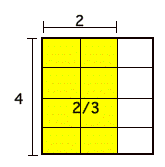 | The fraction 2/3 had two pieces shaded. Each piece was chopped into 4 smaller pieces, which means that there are now 2x4=8 small pieces shaded |
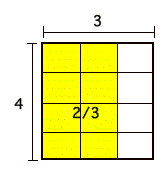 | The fraction 2/3 had 3 pieces in all (or in one whole). Each piece was chopped into 4 smaller pieces, which means that there are now 3x4=12 small pieces in a whole |
Many people also teach equivalent fractions using fraction circles and fraction bars, but in these models, students find common denominators by a guess and check method, and the multiplication property is not as easy to see:
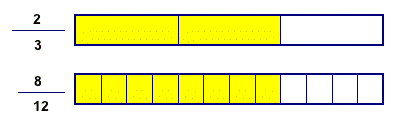
With both of these manipulatives, the teacher needs to choose the problems wisely, epecially when looking for commond denominators. Specifically, problems must be chosen so that it is possible to do the problem: fraction circles and fraction bars come in a limited number of sizes. If you are doing 1/3 + 1/5 and your set does not have 15ths, it would be impossible to solve the problem using manipulatives. I should point out that it is a good thing that fraction circles don't come in too many sizes. If you are comparing by hand it may be hard to tell if 10ths or 9ths is a better fit for finding a common denominator, and this problem would get much worse if you were comparing with, say, 14ths and 15ths.
Discrete model equivalence is rather tricky too, because the whole changes sizes so dramatically. For example, all of these sets are equivalent to 2/3:
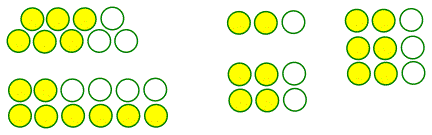
Notice that the three examples on the right are "stacked" (2/3 on top of 2/3 on top of 2/3). This makes it much easier to show the grouping, so that students can visualize that it is equivalent to 2/3:
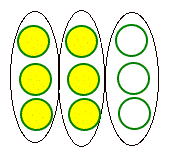 | In this diagram you can see that 6/9 dots are shaded, and that 2/3 groups of dots are shaded |
Being able to make equivalent fractions where the whole is shown as a different size is a valuable skill, but children need time to explore and understand the idea before they are ready to build on it.