1. Explain the difference between a partitive and a measurement interpretation of division.
In both situations, you start with a total amount of something, that you are going to split up into groups. In a partitive division, you know how many groups to split it into, and you need to figure out how many in each group. In a measurement division problem ,you know how many should go in each group, and you have to figure out how many groups there will be.
2. Explain how a child might solve 15 ÷ 3 by direct modeling in a partitive way.
3. Explain how a child might solve 15 ÷ 3 by direct modeling in a measurement way.
4. Explain how a child might solve 15 ÷ 3, thought of in a measurement model, by using addition, subtraction or skip counting
A child could skip count (add 3's) until they got to 15, while keeping track of how many 3's were added: 3, 6, 9, 12, 15--there were 5 groups of 3.
A child could also subtract 3 from 15 until none were left:
15-3=12
12-3=9
9-3=6
6-3=3
3-3=05 threes were subtracted.
5. Explain how a child might solve 15 ÷ 3, thought of in a partitive model, by using addition, subtraction or skip counting. Explain why this is harder than doing it with the measurement model thinking.
A child could make a guess at how many would be in each group. So they might guess that 2 were in each group and skip count 3 groups of 2. Then they would realize that the number was too small, so they would try a bigger number, and they would keep trying numbers until they found one that worked: 5, 10, 15. This is harder than what to do for the measurement division case because with measurement division, you know what number to skip count by and you can keep going until you get to the total. With partition division, you know how many times to skip count, but you don't know what to skip count by, so it's harder to get started.
6. Write a missing number multiplication problem that corresponds to 15 ÷ 3.
3 × _ =15
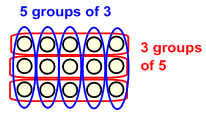
7. Draw a diagram and write an explanation for why 15 ÷ 3 done the measurement way and 15 ÷ 3 done the partitive way will of course have the same answer.

You can put 15 into an array that is 3 down and 5 across, so if you want 3 groups you can look at the rows--then the number in each group is 5--the number of columns, and if you want groups of 3 you can look at the columns--then the number of groups is 5--the number of columns. Either way, the answer is the number of columns, so of course both questions have the same answer.
8. Write a partitive division word problem.
I have personally written rather a lot of these for #10. If you would like me to check yours to see if it's really partitive (and really division), you should e-mail it to me, or bring it to next week's Q&A session.
9. Write a measurement division word problem.
I have personally written rather a lot of these for #10. If you would like me to check yours to see if it's really partitive (and really division), you should e-mail it to me, or bring it to next week's Q&A session.
10. Tell whether each of these word problems is a multiplication problem, a partitive division problem or a measurement division problem. Keep track of which of these you were right on, and make sense to you, and which ones you are finding confusing, and bring your specific questions to next week's Q&A session.
a. Sally has 4 times as many pencils as Jan. Jan has 5 pencils. How many pencils does Sally have? Multiplicative Comparision, Multiplication
b. A pack of Pokemon cards costs $4. If I have $12, how many packs of Pokemon cards can I buy? Cost, Measurement division
c. I have 3 times as many pencils as pens. I have 15 pencils. How many pens do I have? Multiplicative Comparison, Partition division
d. An eraser costs $.12. How much do 4 erasers cost? Cost, Multiplication
e. Ellen has 4 green erasers as 12 pink erasers. How many times as many pink erasers as green erasers does she have? Multiplicative Comparison, Measurement division
f. Ms Anderson has 5 pencil boxes. Each box has 6 pencils. How many pencils does she have? Grouping, Multiplication
g. Four children share 12 apple slices evenly. How many apple slices does each child get? Grouping, Partition division
h. Mark can read 4 pages of his book in 1 minute. How many pages can he read in 5 minutes? Rate, Multiplication
i. I have 10 ounces of cinnamon. If I put 2 ounces of cinnamon in each jar, how many jars can I fill? Grouping, Measurement division
j. Kyle can make 15 paper airplanes in 5 minutes. How many paper airplanes can he make in 1 minute? Rate, Partition division
k. If it costs $12 for 4 boxes of colored pencils, how much does one box of colored pencils cost? Cost, Partition division
l. I can walk 3 miles in 1 hour. How long will it take me to walk 9 miles? Rate, Measurement division