
Polygons are one of the largest named groups of geometric shapes. A polygon is a closed shape, that doesn't cross itself, that is made of straight sides connected end-to-end.

In this list, the words in bold are the ones that are most likely to be introduced first. Words in italics are often used to represent the mathematical vocabulary words in early grades.
A shape can have angles, and each angle can have properties:

Angles within a shape can be compared. Pairs of angles can be described as being:



These words are usually used together to describe a property of a shape. For example, a (carefully stated) property would be: "the shape has at least one pair of opposite equal angles" or "the shape has two adjacent angles that are right angles"

A shape can have sides. The sides of a 2-dimensional shape are called edges. Edges can be straight or curved. The sides of a 3-dimensional shape are called faces. The line where two faces meet on a 3D shape is also called an edge. Faces can be flat or curved. The corner point in an angle is called a vertex (the plural of vertex is vertices).

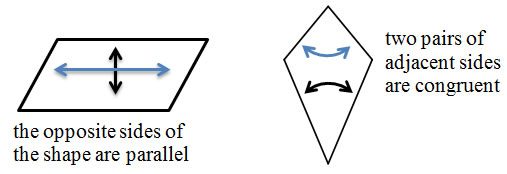
Pairs of sides in a (2-dimensional) shape can be compared. Pairs of sides can be

Examples: "the opposite sides of the shape are parallel", "two pairs of adjacent sides are congruent"
Horizontal and vertical are important words, but they aren't geometric properties that would be used to define shapes because they describe how the shape looks from a particular orientation, and they change if you turn the shape to a different orientation.
Some properties of shapes describe the whole shape and not just a side or angle. A shape can be:



Triangles can be classified by angle size into acute triangles, right triangle and obtuse triangles

| An acute triangle is a triangle where all of the angles are acute. OR An acute triangle is a triangle where the largest angle is acute |
A right triangle is a triangle where one of the angles is a right angle. OR A right triangle is a triangle where the largest angle is a right angle |
An obtuse triangle is a triangle where one of the angles is obtuse. OR An obtuse triangle is a triangle where the largest angle is obtuse |
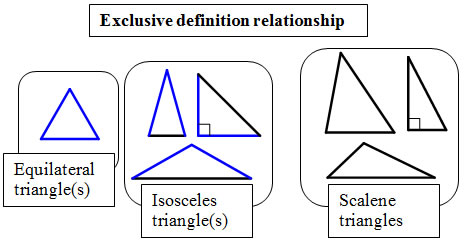
Triangles can be classified by how many sides are congruent. There are two different ways you might find triangles classified:
Scalene always means that all of the side lengths are different.
Equilateral always means that all of the side lengths are the same.
Isosceles sometimes means that at least two of the side lengths are the same, and it sometimes means that exactly two of the side lengths are the same: there's an inclusive and an exclusive version of the definition. I wonder which definition you'll find at your school?

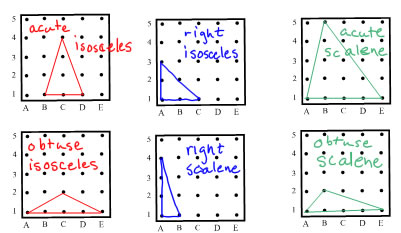
Each triangle can be sorted both by their side lengths and by their angle measures
The Montessori "7-triangles of reality" work involves sorting the triangles in both ways simultaneously into a grid. The children trace the triangles into the grid. In the Montessori work, they use the exclusive definition for isosceles, so the grid looks like this:
Scalene triangles can be acute, right or obtuse
Equilateral triangles are always acute, they are never right or obtuse.
You can make all of the kinds of isosceles and scalene triangles on a geoboard, but you can never make an equilateral triangle on a geoboard (you can get really close, but not quite).
An extra note about geoboards:
When you're looking at measurements on a geoboard there are a couple of mistakes it's easy to make. The first is that when you are measuring lengths, you could be counting dots instead of lengths in between. The length should be the number of spaces (think of each space as an inch), not the number of dots. The other thing to watch out for is that you can't just count diagonally to find diagonal lengths. The distance between two diagonally adjacent pegs is greater than the vertical or horizontal distance between two adjacent pegs. (It's not a simple fraction to tell how much longer, either--you need the Pythagorean theorem for that!) |
 |
Quadrilaterals
Polygons that have 4 sides are quadrilaterals. There are even more named varieties of quadrilaterals than there are varieties of triangles. All of the shapes in this image are quadrilaterals

One property that quadrilaterals can have is that they can have parallel opposite sides. If each side has a parallel opposite side (two pairs of parallel sides), the shape can be called a parallelogram. Everything in the green loop is a parallelogram. Rectangles, squares and rhombuses (rhombi) are parallelograms because their opposite sides are always parallel. It's also correct to say that a quadrilateral is a parallelogram if its opposite sides are the same length, but usually in the definition we use that the opposite sides are parallel because the word parallel is part of parallelogram.
A rhombus has all 4 sides equal length. O is a rhombus, and it's sneaky, but the square P is a rhombus too. (Squares get to be a lot of different things besides just squares).
Quadrilaterals that have one pair of parallel sides are called trapezoids. M, N and U are all trapezoids. Some math books (and lots of mathematicians) say that a trapezoid has at least on pair of parallel sides, so that would make a parallelogram (with two pairs of parallel sides like K, O, P and Q) just a special kind of a trapezoid. Other math books (includingmost of the high school geometry books I've looked at recently) say that trapezoids have one and only one pair of parallel sides. Because words get their meaning from what groups of people agree that they mean, there isn't a right or a wrong definition of trapezoid.
Sometimes we give special names to other shapes too. R, for instance, is a kite because it has two pairs of sides that are adjacent (next to each other) that are the same length. Like "trapezoid", there isn't just one definition of "kite"--some people would say that L is also a kite because it has two pairs of adjacent sides that are the same length, and other people would say it is not a kite because it's concave.
Every triangle can be classified by its angles and by its side lengths, but there are some quadrilaterals that don't have any special classification at all. H and I, for example, aren't part of any named group of quadrilaterals.
One important idea about classifying shapes is that the same shape can fit into more than one category, and some categories are subsets of other categories. For example:
| A square is a special kind of rectangle. All squares are rectangles. Some rectangles are squares, and some rectangles are not squares. | 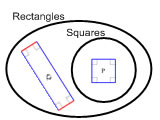 |
| A square is also a special kind of a rhombus. All squares are rhombi (or rhombuses). Some rhombi are squares, and some are not squares. |  |
| A square is a rectangle and also a rhombus. Everything that is both a rectangle (90° angles) and a rhombus (all equal sides) is a square. |  |
| Squares, rectangles and rhombi all have parallel sides, so they are all parallelograms. There are some parallelograms that don't fit rectangles, rhombi or squares. | 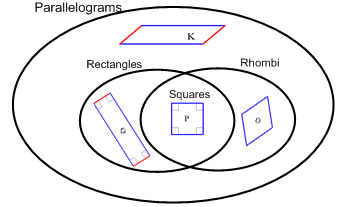 |
All of these shapes (as well as trapezoids, kites, and a few others) are quadrilaterals because they all have 4 sides.