
Rate and price problems (introduction):
This table summarizes the different kinds of problem types listed on page 48 of Children's Mathematics:
In the previous lesson, we looked at grouping problems. In this lesson, we'll be looking at rate and price problems. Rate and price problems are very similar, and neither is particularly more difficult than the other. More significant is that measurement division problems are more difficult than multiplication, and partitive division problems are more difficult than measurement division. An interesting resource I found is a set of multiplication and division word problems created by South Dakota Counts. If you look through those problems, you'll see that they recommend using multiplication and measurement division rate and price problems in second grade, but they don't include partitive division rate and price problems until third grade.
Rates can be phrased in many different ways:
The first thing you think of when you hear "rate" is probably miles per hour. There are some more and less formal ways of expressing miles per hour, though. Each of these is a different way of giving the same rate for a word problem. You'll probably recognize that some of these will be easier for younger children to decode than others:
Rates don't have to be miles per hour, however. They can also compare other amounts:
Be aware when you write your own rate problems, that a rate is technically something of the form _____ per ____, or ____ for each _____. So, if you're describing your rate as 6 miles in 2 hours rather than 3 miles in one hour, then you're making the problem more complicated (always start with simpler problems and then introduce more complicated ones after children have experience with the simple ones).
Price statements are really similar to rate statements. In fact, a price is a special kind of a rate: a price per item rate:
Cuisenaire rods:
Cuisenaire rods are rods, the smallest of which is 1cm x1cm x1cm. All of the other rods can be mesured using white cubes. We introduce Cuisenaire rods to encourage children to think about numbers as a unit rather than as countable units (often these are introduced in second or third grade). Typical ways to use Cuisenaire rods it to make a long train of rods to show a total length, and/or to compare totals by putting trains side by side to compare the lengths. Most Cuisenaire rods are unmarked. I use marked ones on the computer because it's really hard to line up unmarked ones with each other on a computer screen. |
 |
Examples of rate problems, and how to solve them using Cuisenaire rods (note--all of these problems can also be solved by counters. I just am using this lesson to also introduce Cuisenaire rods as a teaching material):
multiplication:
Aubrey walks 3 miles an hour. How many miles does she walk in 5 hours?
Direct modeling solution:
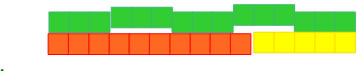
(note a more typical solution would have the rods all in a straight line--I just offset them so you could see the individual rods)
measurement division:
Tyler can ride his bike 6 miles in an hour. If he lives 18 miles from town, how long will it take him to ride to town?
Solution process:

partitive division:
Susan jogs 12 miles. It took her 3 hours. If she jogged the same speed the whole distance, how far did she jog in one hour?
Solution process:

Examples of price problems and how to solve them using Cuisenaire rods.
multiplication:
A pair of headphones costs $5. How much do 4 pairs of headphones cost?
Solution process:

Partitive division:
A pen costs 12 cents. Molly has 48 cents. How many pens can she buy?
Solution process:
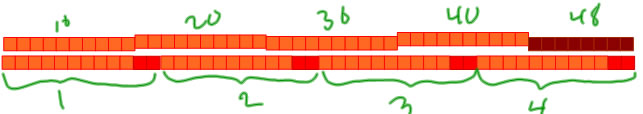
Comparison to grouping problems:
multiplication:
Gropuing: A box of crayons has 8 crayons in it. How many crayons are in 4 boxes?
Rate: Aubrey walks 3 miles an hour. How many miles does she walk in 5 hours?
Price: A pair of headphones costs $5. How much do 4 pairs of headphones cost?
In a multiplication problem, the multiplicand (amount in each group) and the multiplier (number of groups) are both known. In a rate problem, the rate plays the part of the multiplicand, and the amount compared to by the rate (per hour) plays the part of the multiplier. In a price problem, the price per item plays the part of the multiplicand, and the number of items is the multiplier.
multiplication problems are solved by showing the multiplier number of sets, with the multiplicand in each set, and finding how many in all.

5 sets of 3: 5 is the multiplier, and 3 is the multiplicand.
measurement division
Grouping: Karen had 12 apples. Her apples were in bags, and each bag had 3 apples. How many bags of apples did she have?
Rate: Tyler can ride his bike 6 miles in an hour. If he lives 18 miles from town, how long will it take him to ride to town?
Price: A pen costs 12 cents. Molly has 48 cents. How many pens can she buy?
In a measurement division, the total (product) is known, and the amount in each group is known (multiplicand). In a rate problem, the rate (unit rate--rate per something) takes the place of the multiplicand, and the total is the product. In price problems, the multiplicand is the price per item (price for 1/each item), and the product is the total price. Note that measurement division problems, because the unknown is the number of groups, are the only multiplication or division problems that really compare apples to apples (or miles to miles or cents to cents) rather than apples to bags.
Measurement division problems are solved by building the total amount of the product, and measuring it using repeated groups (with the size given by the multiplicand). The answer to a measurement division problem is the number of groups

18 ÷ 6: 18 is the product, and 6 is the multiplicand
Partitive division:
Grouping: Karen had 12 apples. Her apples were in 4 bags, and each bag had the same number of apples. How many apples were in each bag?
Rate: Susan jogs 12 miles. It took her 3 hours. If she jogged the same speed the whole distance, how far did she jog in one hour?
Price: Jeremy bought 4 boxes of modeling clay. He spent $24 in all. How much did each box of modeling clay cost?
In a partitive division problem, the total (product) and the number of groups (multiplier) are known. In a rate problem, the product is the total, and the multiplier (number of groups) is the amount compared to or measured by (hours); note that the total and the number of groups are different kinds of measurements: apples and bags, miles and hours, dollars and boxes. In a price problem, the product is the total amount of money, and the multiplier (number of groups) is the number of items purchased.
To solve a partitive division, the total is built, and then by guess and check a size is found that you can use to measure the total using the given number of groups.

12 ÷ 3: 12 is the product, and 3 is the multiplier. It tells the number of groups. The size of each group must be found by guess and check.