A sample dot pattern for 7=4+3 is
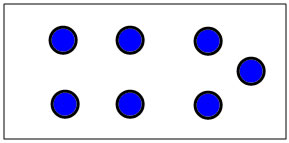
A sample dot pattern for 7=5+2 is

1. You should know and/or be able to invent on the spot dot patterns and 10-frame patterns that show numbers between 5 and 10 decomposed in different ways. For example, you might be asked to show a dot pattern that shows that 7=4+3, or 7=5+2.
A sample dot pattern for 7=4+3 is
|
A sample dot pattern for 7=5+2 is
|
2. You should know the most common decompositions for the dot and 10-frame patterns from the lecture.
a. For example, if you were asked to list some decompositions that a child might suggest for the pattern:

you should recognize that the most common decomposition here is 5+3. Another likely decomposition here is 6+2.
b. And if you were asked to list some decompositions that a child might suggest for the pattern:

you should recognize that the most common decomposition here is 4+3. Other possible decompositions here include 6+1 and 2+2+3.
3. You should be able to give at least two examples of where students might subitize quantities (showing numbers on fingers, 10-frames, dot patterns), and you shoule be able to explain what the word subitize means (to recognize how many without counting).
4. You should be able to come up with at least 4 questions that one could ask about a dot pattern or a Cuisenaire rod comparison:


5. You should be able to write at least 5 versions of a number sentence corresponding to the above Cuisenaire rod comparison.
6. You should be able to explain what it means to compose and decompose amounts.
Composing means to put together two amounts to get the sum.
Decomposing means to see an amount as being made of (two) smaller amounts--to split it into smaller pieces.
7. You should be able to describe several ways of teaching and practicing decomposition of numbers below 10.