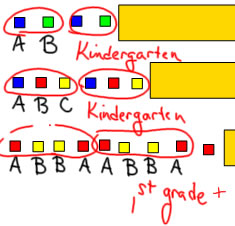
K-1 patterns
When children first work with patterns, they work with repeating patterns where the pattern is fairly short (usually 2 or 3 in length) and not too tricky. For example, the AB and ABC patterns are simple enough for kindergarten children to understand, but the length 4 ABBA pattern is extra tricky (because it begins and ends with the same thing) and it's a bit long--it's harder to hold a pattern of length 4 or 5 in your head than a pattern of length 2 or 3.

When you're showing a pattern to children, it's important to show enough for them to be confident that they know how the pattern goes. When working with repeating patterns a good rule of thumb is to show at least 2 full repetitions.
Children in kindergarten can understand patterns well enough to tell what comes next in a pattern, to build onto a pattern and to make repeating patterns of their own.
Questions about repeating patterns (patterns in grades 1-3)
An activity to get children thinking about what comes next in a pattern is to make a pattern with counters and cover up some of the counters with cups or something similar. Leave at least the first two repetitions uncovered and ask what comes next? or point to a cup and ask: what color is under this cup? Children in K-1 can usually figure out the hidden counter by pointing to or touching the cups and reciting the pattern as they go:

By second grade, children can figure out the hidden counters for a few steps beyond the end of the cups. An AB pattern like this one is easier, of course to figure out because the colors correspond with odd and even numbers. With an ABC pattern or this AAB pattern, it's more complicated, because you need to know things like multiples of 3:
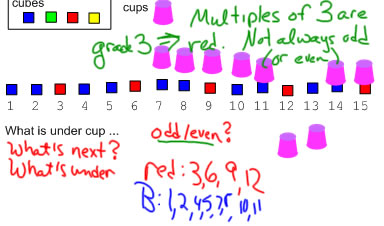
Repeating patterns can make a good bridge to skip counting for children in grade 3 and 4. With an ABC pattern like this (or any pattern where each letter is used only once) the question of which numbers go with a given color produce numbers in a skip count pattern:

By giving a pattern to follow for the skip count sequence, it ties skip counting to a something more concrete. Children have a more concrete (draw the sequence) and a more abstract way of finding the sequence of numbers (skip counting or repeated addition). By extending the amount of the sequence you ask for with numbers beyond the spaces for the pattern on the page, it encourages children to find and use the more abstract pattern, while giving them a visual way to understand what the pattern is and does.

By asking for the skip count patterns for different shapes in a jumbled up order (star, sun, raindrop, moon rather than the order they appear in the pattern), it encourages children to keep using the order of adding 4 as they go across rather than adding down in the columns. (There are other learning goals for which it would be a good idea to use the order that the symbols appear in the sequence)
This skip counting skill and strategy is important in grade 3 and 4 as a way to understand and compute multiplication.
Skip counting skills, once they are introduced and understood can be effectively practiced in more abstract contexts. Some examples are...
| the sorts of skip count problems you find on standardized tests: |  |
| or skip count puzzles: |  |
Children can recognize and continue patterns that are simple enough for them to understand.
Making predictions about what comes next and what comes later in a pattern develops reasoning skills for understanding, representing, and relating numbers to patterns.
Patterns can give children a concrete way of thinking about skip counting and other numerical patterns.
Skip counting is an important skill for children to develop and reason about in third grade.
As children practice skip counting in a variety of contexts, they develop and extend their understanding of multiplication.