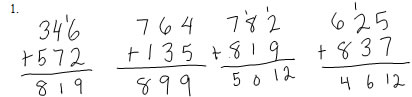
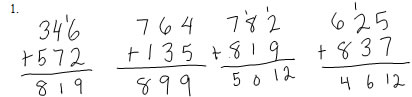
This is an error pattern.
It's most clear that something's going wrong in the third example, where there is a 12 in the one's column.
If you look at the first example there is a 1 "carried" into the ones column. That's another thing that shouldn't happen. The column just to the left has a sum of 11, so the student has put one of the 1's in the tens column and one is "carried" to the ones column. We can't tell from this example, which digit is being "carried". We can tell, however, that the student is working left to right because the 1 in the ones column had to have come from the column to the left.
Looking at the third example, the 1 above the 8 in the tens column will have come from the hundreds column if the student is doing things consistently. 7+8 in the hundreds column yeilds 15, and the 1 was "carried" to the tens column.
This is a student who knows the steps of the standard algorithm reasonably well, but has reversed the order from right to left to left to right. A discussion with the student might tell you whether they understood why numbers have to be exchanged to move amounts to the column to the left. If they do understand that, then your task as a teacher is only to reconnect their knowledge about trading to their knowledge of the number algorithm. If they don't understand the basics of trading, then you should be looking for significant remediation opportunities that can involve the student.
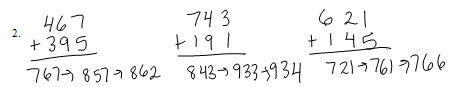
This yeilds correct answers, and is an alternate algorithm. You should recognize this as a mental arithmetic algorithm you saw in a previous lesson. In this algorithm, the place values in the second addend are added sequentially to the first addend.
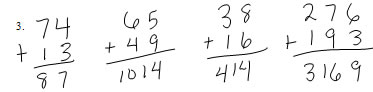 '
'
This is an error pattern you should recognize from the lesson. The student who is solving these problems is adding the digits in each column, and is not trading so that values end in the correct place value. This student probably needs more practice with trading between place values. If the student understands trading with manipulatives, then he or she needs practice connecting that knowledge to the numerical algorithm.
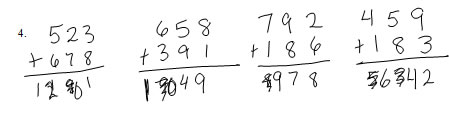
This yeilds correct, if awfully messy answers. It is an alternate algorithm.
The scribbled out numbers are easiest to see in the fourth example. You can see a scribbled out 5 in the hundreds column that is replaced by a 6, that means the the sum of the hundreds was done before the tens column, so the student must have added 400+100 to get 500 before adding 80+50=130, and combining 100 from 130 with 500 to get the final 600. That also works with the tens place where you see a scribbled out 3 that's replaced by a 4, so the 3 comes from 130, and it's changed to a 4 after 9+3 is added to get 12, and the 10 from 12 is combined with the 3 in the tens place to get 4 in the tens place.
If you look through the other examples, the same process explains the numbers there. For example, in the first example, if you started with adding 500+600 to get 1100, and it looks like there was a 1 in the hundreds place that was written over by a 2, and 20+70=90, accounting for the scribbled out 9 in the tens place, which is fixed up after 3+8 is added to get 11, and the 10 is combined with th 9 in the tens place to get 10 tens, which then gives 100 to combine with the 1 in the hundreds place to get you the written over 2.
This algorithm follows what you would do with manipulatives if you started by combining the hundreds first.
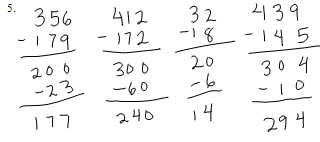
This works. It is an alternate algorithm. It's a different way of writing down the negative numbers algorithm. The student subtracts in each column to create a new minuend and subtrahend. If the resulting number is positive, it goes in the minuend, if it's negative, it goes in the subtrahend, then the difference of the new numbers is computed mentally.

If you saw only the second example, it would look like this student was subtracting correctly, but in the other examples, you'll see that there are two digits in the ones column. Looking at the first example, the student correctly exchanged a ten for 10 ones, and subtracted 18-4 correctly, so what went wrong? Well, they shouldn't have exchanged at all, since 4 is less than 8. The student is trading tens for ones whether or not it is needed.
This may even be a rare example of an error that would be easy to correct simply by pointing out the problem to the child. If it isn't fixed quickly, you'll want to look for further misunderstandings.
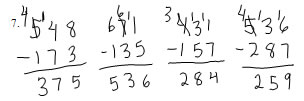
The first example is correct. The second example is correct. The third and fourth are not. What's going on? When the child has to "borrow" once, they do so correctly, but when two exchanges are needed, they are making an error wherein they trade in a hundred and take 10 tens and also 10 ones without trading in a ten.
It looks like this child is trying to do some variation on the trade first version of the standard algorithm, but they are performing a set of steps that "look right" to them, without thinking through the meaning of the trades. In working with this student it might be effective to discuss how 1 hundred = 10 tens which makes the trade in the first example a fair trade; and 1 ten = 10 ones, so the trade in the second example is a fair trade, but 1 hundred isn't the same as 10 tens and 10 ones, so that's not a fair trade.
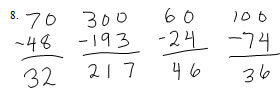
It looks a bit like this child is subtracting mentally. Most of the answers are off by 10. It's not clear whether they are trying to subtract mentally, and whether they are subtracting right to left or left to right. The answers could be explained (and replicated) by treating almost all of the 0's as 10's--the only exception is the fouth example, where 7 tens is subtracted from the 100=10 tens that is written, and not from 100 and another 10 tens.
A discussion with this child would be most helpful to determine just how they're thinking about things to get the answers. Some children do strange things when there are 0's in the problem, in which case this would be a very quirky error, and probably quite difficult to correct. Other children could reach these answers by subtracting mentally, and not compensating for the trades--those children might be well served by thinking through subtracting one place value at a time. For instance, in the first example, the teacher could ask what's 70-40, and then following it by 70-48.

And here we have the alternate algorithm where you mentally subtract one place value at a time. This student has recorded each of the partial differences, and circled the final answer.
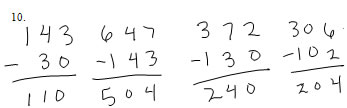
None of these problems require trades, so that isn't the problem. Example 2 and 4 are fine. In example 1 and 3 there are some mystery 0's. Why do we have 3-0=0 and 2-0=0? Apparently this child has some confusion about 0's--it might be a confusion with the multiplication rule: 0x(anything) = 0, or it might be something harder to track down, but apparently this child is consistently thinking that: anything - 0 = 0. You'd think this would be an easy error to correct, but in my experience it comes as part of some deep seated misunderstandings and is surprisingly resistant to change.