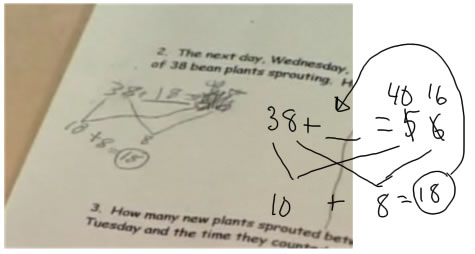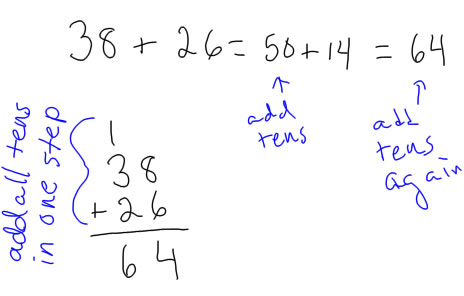Lesson 2: Teaching 2-digit addition and subtraction by questioning and discussing.

Overview:

2-digit addition and subtraction is a key concept and skill for second grade. Children will have some experiences with it in first grade, and will continue to build on it in third grade, but the bulk of the teaching and learning happens in second grade. When teaching 2-digit addition and subtraction using a constructivist teaching approach, it's important to be very clear in your own mind what the important goals are for children to reach.

• Children need to develop strategies that are reliable for adding and subtracting two digit numbers--they need strategies that make sense and will produce the correct answers.
• These strategies should be efficient in the sense that they use the base 10 structures of numbers--children should be combining or separating tens, and ones. (Children should move past strategies that consist of counting on or counting back by ones)
• Children need to have efficient ways of recording their work on paper.

These goals are roughly in order of importance and of progression--later goals build on earlier goals.

The main tasks of a teacher are to

• ask appropriate problems
• facilitate children's sharing of strategies
• facilitate children's analyzing their peers strategies
• encouraging clear ways of recording the strategies.

In order to facilitate discussions and help children record their strategies, it's helpful to know some of the strategies children often come up with to solve probems.

It's tricky to know what to do about the standard algorithms when you are teaching in this way.

Children need good strategies

When children solve problems that make sense to them in ways that make sense to them, the method they use for solving is their strategy for solving. To learn addition and subtraction with 2-digit numbers, children need good strategies for solving those types of problems. The first criterion for a good strategy is that the child be able to use it to find correct answers.

We want more than that to happen, though, we also want children to have strategies that are efficient, and will provide a good background for later work they will need to do, so we also need children to learn and develop strategies that use place value. Being able to take away using tens and ones, rather than just one at a time is a great advantage.

Finally, children need to be able to record their work in a way that they can use to show someone else what they were doing, and in a way that helps them keep track of the steps of the problem. Being able to transfer some of the mental work of adding and subtracting to the paper from your brain is a good thing, that lets you solve more complex problems.

Teachers must ask appropriate problems:

Problems should be of a variety of problem types. It's especially valuable to have not only separate subtraction problems, but also part unknown (PPW-PU) problems, and comparison problems. The problems should be presented in a familiar context that children understand, and that make it easy to visualize and understand what the problem is asking.

Problems should use appropriate numbers at each stage. Children should begin with adding and subtracting numbers where regrouping (trading, exchanging) is not required, for example adding 34 and 52 or subtracting 34 from 79. A goal of adding and subtracting with numbers that don't need regrouping is for children to add and subtract by joining or separating tens and ones separately. At this stage, the task of adding and subtracting shouldn't be simplified for them: by this I mean that this is not good timing to teach children to line up columns and add in each column. That recording strategy is a good one, but it disguises understandings and misunderstandings, and its easy to believe that children understand more than they really do (for instance, if you line up 24 and 62 and add, and if you say to yourself: 4+2=6 and 3+5=8 so the answer is 86, are you really remembering that the 3, 5, and 8 are numbers of tens, or are you thinking of those as ones?)

Once children understand adding and subtracting without regrouping using tens and ones, then its time to start adding and subtracting with regrouping, so again, the teacher poses questions, this time ones where regrouping is needed. Usually you ask questions that require regrouping in addition first: 37+44 or 39+8 and 39+18. There are a couple of ways to ease into these problems.

• Using manipulatives: You should plan ahead for this one, and during the first weeks of second grade, children should have a chance to do regrouping activities and games that are not overtly addition and subtraction (like those discussed in the lesson on base 10 manipulatives). Then, when it comes time to add and subtract using regrouping, children have a familiar material to work with. One way to use manipulatives here is to first ask children if they can figure out a problem with regrouping (eg. 36+28) using the manipulatives. When children have had a day or two to solve problems using manipulatives, you can start asking--how can we write down on paper what we're doing with the manipulatives. From there, you may wish to go on to more open ended work: add 38+47 in any way that you want. Explain how you did it.
• Using a context that naturally groups into tens and ones. In the article: Second Graders Circumvent Addition and Subtraction Difficulties, the teacher uses the context of her Aunt Mary's candy making, where she packages the candies in groups of 10. This context helps children think in terms of tens and ones as they represent the solution process.
• Using the make 10 strategy as a jumping off point: when the class is practicing make 10 to add, and they are adding 9+7 by making a ten, they can practice that strategy also by adding 39+7.

After children have developed good strategies for adding with regrouping, it's time to practice subtraction with regrouping. A good place to start with subtraction is numbers where you are taking away a 1-digit number from a number of tens, eg. 30-6. Breaking down a single ten from 30 is easier than breaking down a ten in 31, so problems where the minuend (the number you are subtracting from) has a 0 in the ones place is a good place to start. Moving on from there, problems are more difficult when you are subtracting a 2-digit number and they are more difficult when the ones place of the minuend is not 0. The teacher should be creating problems that slowly get more difficult.

Teachers facilitate children sharing their strategies and analyzing each others strategies

As part of most problem solving lessons, children should be sharing not only what answers they found, but what they did to find the answers. The children and teacher all need to think carefully about the strategies that children are sharing. The teacher should help this thinking and understanding by asking questions that will make the strategy more clear to the class. The teacher can also facilitate by asking another student to rephrase or re-explain a strategy shared by one of their peers. It's helpful as a teacher to be familiar with some of the kinds of strategies children often discover, but the most important skill to have is to be able to listen carefully and understand the strategies children share, and be able to make sense of them.

This facilitation step is a great tool for the teacher. Sometimes teachers ask the whole class to try to understand and use a strategy shared by one or two of the students as a way to get the class to develop shared strategies for solving problems.

Teachers encorage clear recording strategies:

One of the places where a strictly constructivist approach sometimes leaves gaps is that some children will choose to do all of their arithmetic mentally, and they won't have an efficient way of recording the strategies they are using. This is somewhat problematic in places where they have to communicate their thinking to others. This is also problematic when children encounter very complex problems, because mental arithmetic strategies require you to remember and track several steps mentally, whereas a good recorded strategy lets the paper keep track of part of the work. This is find for most problems, but when you encounter a bigger or a more complicated problem, then you need to be able to reduce the strain on your memory by off-loading some of that work to the paper, so having a good way of recording the thinking steps of the strategy as well as the answer can be a real advantage.

Guiding students to develop and adopt such a recording method involves spending part of the strategy discussions on things like: how can we show on our paper that we regrouped to make a 10, or how can we show that we traded a ten for 10 ones?

Teachers think about strategies that children use for adding and subtracting

Addition strategies:

Addition is much more straightforward than subtraction in many ways, and the variety of problem solving strategies is fairly small. When adding a two digit number that involves regrouping, such as 38+17, the main strategies you will see are:

• Counting on by ones: a most inefficient strategy, but you will find children who will count on 17 from 38 to add the numebers.
• Conting on by tens and ones: A child might say: 38, 48, 49, 50, 51, 52, 53, 54, 55 (Much better as counting on strategies go. This works well on a 100-grid)
• Adding the tens, then adding the ones from the first addend, and then the ones from the addend: 38+10=40; 40+8=48; 48+7=55.
• Adding the tens, and the ones and then combining: 30+10=40; 8+7=15; 40+15=55.
• Adding the ones and then the tens and then combining (this is the standard algorithm. It's a refinement of the previous strategy. This is slightly more efficient than the previous strategy, and is pretty rare except among children who have been taught the standard algorithm)

Subtraction strategies:

There are a lot of subtraction strategies. Please watch the Bean Sprouts video carefully (it's number 15), and see if you can figure out how children are thinking about the problems. You can print out this sheet to take notes on. When you've watched that video, come back to thie page and read my ideas....

This child is using what is sometimes called the negative numbers strategy for subtraction. If you are taking away 7 ones from 6 ones, one way to think about that is that you get -1, which means you have 1 more left to take away. With this strategy, you subtract in each place value, but when the subtrahend digit is greater than the minuend digit, then you must record the difference as a negative number, which you can think of as an amount remaining to be taken away. After subtracting in each place value, you must combine those place value answers using either addition or subtraction as appropriate to get the final answer.

This child is counting back by ones.

This child is doing the closest thing to the standard algorithm of all of the children in the class. He is trading a ten for 10 ones to make 50+6=40+16. This looks unfamiliar to you because he is thinking of the problem as a missing number addition problem, rather than as a subtraction problem. After he regroups he is asking what can I add in this place value to get the total? So he thinks 30+?=40, and gets 10; and he thinks 8+?=16, and gets 8. Those missing addends are equivalent to the difference you get if you subtract: 40-30=10 and 16-8=8. Finally he adds the two parts of his missing addends to get 18.

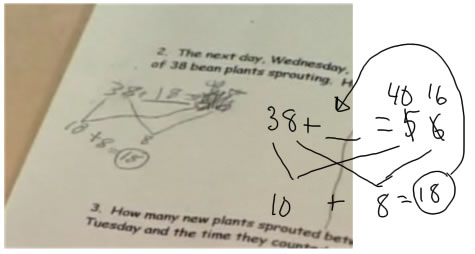This child is counting by ones to find the difference. This representation is a direct modeling representation.

Two boys accidentally added on the third problem. What does their work show you about how they are adding? In the left example, the boy is adding in the tens place and in the ones place, and then combining those partial sums. In the right example, the boy is also adding in the tens place and in the ones place. His representation is similar to that in the standard addition algorithm because he is recording the 1 ten from 15 in the tens column, but he appears to be doing this after he added in the tens column, and then he is combining those answers to get his final sum.

This boy is using a common mental arithmetic technique for subtraction. He is subtracting the tens first and then compensating step by step by figuring out how the ones place value changes the solution. The number 7 in the ones place comes from the subtrahend, so it has to be subtracted to get to the final answer. Remembering that subtracting from a multiple of ten (a number with 0 in the ones place) is easier for most children, so that's probably why he is choosing to work with the 7 first. The number 6 in the ones place comes from the minuend, so it has to be added to get the final answer, so that last step is to add on 6.

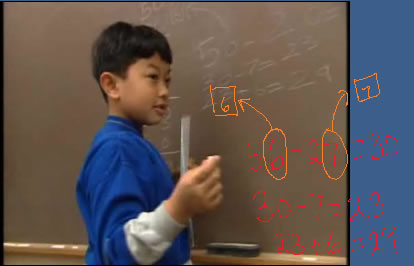This problem is very similar to the previous one in that it is a mental arithmetic strategy, and it breaks up numbers by place value. This girl is breaking up only the subtrahend, and then she is mentally subtracting by subtracting first the tens and then the ones. You can see here, I think, that subtracting 8 from 26 is somewhat harder than subtracting 7 from 30 was in the previous problem. This strategy has fewer steps, but it requires more mental work than the previous one.

If you choose to teach addition and subtraction by posing and discussing good problems, you are likely to see most of these strategies being used by your students. As a teacher, you try to track each of your students, and help them find efficient strategies that make sense to them, and you help them find good ways of recording their strategies to share with others.

What about the standard algorithms?

The standard ways we all learned to add and subtract in school have some real advantages. They're efficient, they fit into a small amount of recording space, and they flow nicely with other algorithms for other things like multiplication and division. So. Do children need to learn them? If so, when should they learn them? If that's the first thing that they learn, then maybe they miss out on the richness of all of these other problem solving strategies. If they don't learn them, then maybe they'll get into another class next year and be lost because they don't have the same background as everyone else. It's quite a dilemma. Different people make different claims about this--some claim that children shouldn't be taught the standard algorithm at all, instead they ought to have a set of other strategies that they choose from depending on the problem. Others claim that it's important to learn the standard algorithm and practice it to the point of automaticity--to the point where you can do it without thinking about it, and so free up brain space for other things.

I think it's possible to have a middle ground approach to the question: it's possible to introduce the standard algorithm at a point after children have spent a reasonable amount of time becoming comfortable with coming up with their own strategies. Ideally, the standard algorithms should be tied into a student-created strategy that is similar. If you look at the addition work from the two boys in the beans lesson, you'll see that their addition strategies: add tens and add ones and then combine, is quite similar to the standard algorithm. At some point the standard addition algorithm can be introduced as a variation that people have found to make that strategy a little more efficient. The standard algorithm is just a little more efficient than the strategy where you add the tens first and then the ones, because you only have to add the tens once. If you add the tens first, then you end up adding tens twice instead of once:

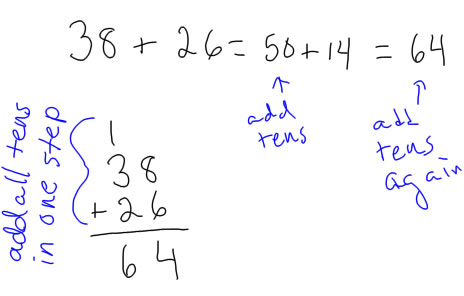
With subtraction, it would be great to have the missing number addition strategy to compare to:

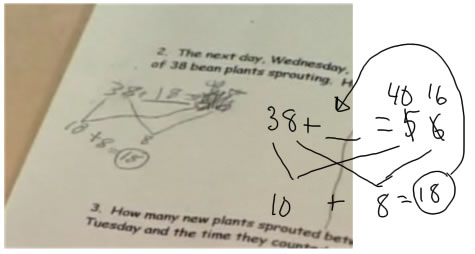
The standard algorithm is very close to this--indeed it's doing exactly the same thing--but if you write it as subtraction instead of addition, you can make the problem fit together in a more compact way, so it's essentially a more compact way of recording the same work:

I am personally suspicious of the extremes of any argument, so I would probably try to reach a middle ground like this in teaching second grade. I think at the moment the research on both sides is weak enough to be inconclusive. That may change in the future, however, so you should be aware of the options you have as a teacher, and reflective in your practice of what you see working for your students.